How Squat, Bank and Bank Cushion Effects Influence Ships in Restricted Waters?
When a ship moves through restricted waters, it has to navigate close to the shore and other manmade structures because of limited navigable width. The shallow water and proximity of the sides of the channel effects the ship navigating through the restricted waters. These effects cause errors in maneuvering which can lead to grounding or collision.
Any ship (regardless of its size) navigating through restricted waterways is heavily affected by hydrodynamic effects. In this article we will understand three of the most common effects experienced by ships – Squat, Bank and Bank cushion effects.
Squat effect: When a ship moves through the shallow water, some of the water displaced rushes under the vessel to rise again at the stern. This decreases the upward pressure on the hull, making the ship sink deeper in the water than normal and slowing the vessel. This is known as squat effect, which increases with the speed of the vessel.
Bank effect: Bank effect refers to the tendency of the ship’s stern to swing towards the near bank when the ship is operating in a river or restricted waterway.
Bank Cushion effect: When the ship is near to the bank, the water is forced between the narrowing gap between the ship’s bow and the bank. This water tends to pile up on the starboard side of the ship, causing the ship to sheer away from the bank.
Before we move ahead, let’s understand some of the basic principles of physics we will be using to understand these effects.
Consider a horizontally placed hourglass-shaped transparent cylinder. Let us make an arrangement wherein water passes through that cylinder which is partially constricted at the middle as shown in figure 1.
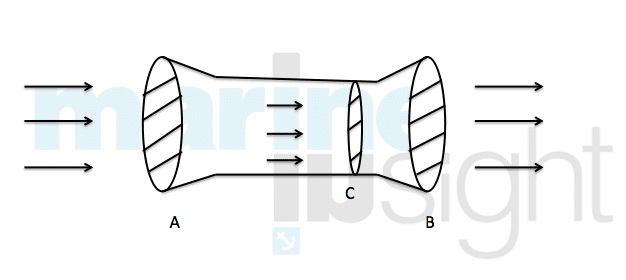
What do you notice?
You will notice that the liquid flows at a faster pace in the constricted area ( fig1).
Why does this happen?
There is an equation in physics that is known as “ Continuity Equation” which states that when a fluid is in motion, it must move in such a way so that the mass is conserved. This means that in a continuous flow of fluid in fig 1, the mass of fluid passing through point A is equal to that at B and also at C, in unit time.
Clearly, the mass of the fluid passing through the cross section (shaded area) at point A will be equal to that passing through point C or B.
To make this happen, the rate of flow of water at constricted point C must be more than that at A or B. This also means that the velocity of flow at a constricted point is always greater so as to satisfy the Continuity Equation.
Theoretically, if A is the cross section of the cylinder and v is the velocity of an inviscid (non sticky) fluid, then A is inversely proportional to v, i.e., Av = constant.
Now one may ask why we are delving into all these when the topic is Squat effect and Bank effects on ships passing through restricted waters? Simple, to clearly understand these effects we will have to realize a few basic principles.
The next is Bernoulli’s Principle, which states that for an inviscid flow (flow of an ideal fluid that has no viscosity), an increase in the speed of the fluid (i.e. its Kinetic Energy) occurs simultaneously with the decrease in pressure or decrease in its Potential Energy and vice-versa. Actually this principle is a corollary of the Law of Conservation of Energy which says the sum total of all the energies in an isolated system (roughly speaking no energy can be transferred into or out of this system) always remains the same
Thus in our first experiment (fig 1), the increased speed at constricted point C caused a decrease in pressure at that point. If you want to see an experiment you can watch the video below:
Thus, theoretically, if P is the pressure at a point and v is the velocity of an incompressible fluid, then:
v = 1/P
The Squat Effect
Without going into anymore mathematical complexities, let us now understand the Squat Effect.
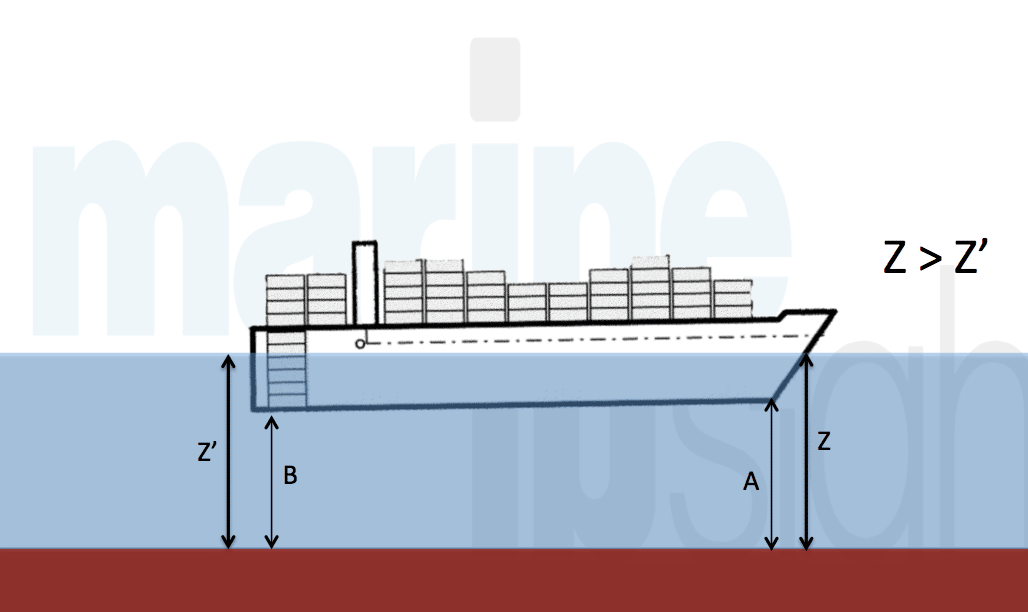
Let us consider the figure 2 of a ship in restricted waters:
A ship S is plying in shallow water.
If z and z’ be the underwater clearance at fore and aft respectively, then obviously from the diagram it is evident that z> z’. Thus clearance under the keel at point A is greater than at point B. Now from the Continuity Equation we know that velocity of the flow of water (it is considered to be non-viscous and incompressible) at the stern is greater than that of the bow, or simply the flow is asymmetric.
But Bernoulli’s principle tells us that with increase in speed of flow at B, there is a decrease of all round pressure at B, which means that at B (at the stern) the ship will sink further and may touch the channel bed. If the vessel is trim by head, the bow will tend to sit in the mud because of less pressure. This is the Squat Effect.
Squat effect increases doubly with speed. If the ship sinks x cm due to Squat Effect due to y speed then it will sink 4x cm in 2y speed.
Thus to minimize squat, the pilot or the master of the ship has to maintain slow speed. If the pilot sees that with no considerable change in shaft speed, the vessel is slowing down, then the Squat is happening. He should immediately reduce the speed to minimize the Squat effect.
Bank Effect
Till now we have considered the keel’s proximity to the river or channel bed. Now we will consider the hull’s proximity to the bank. Let’s refer to the figure 3.
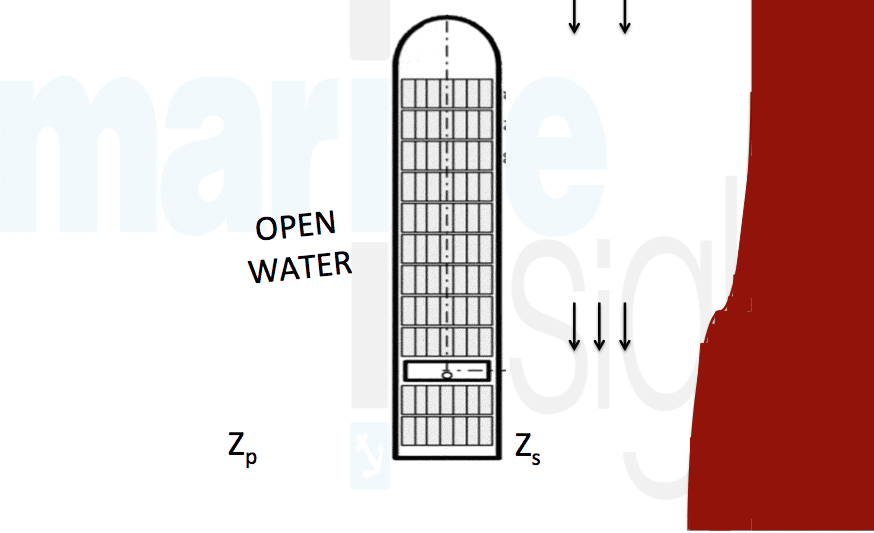
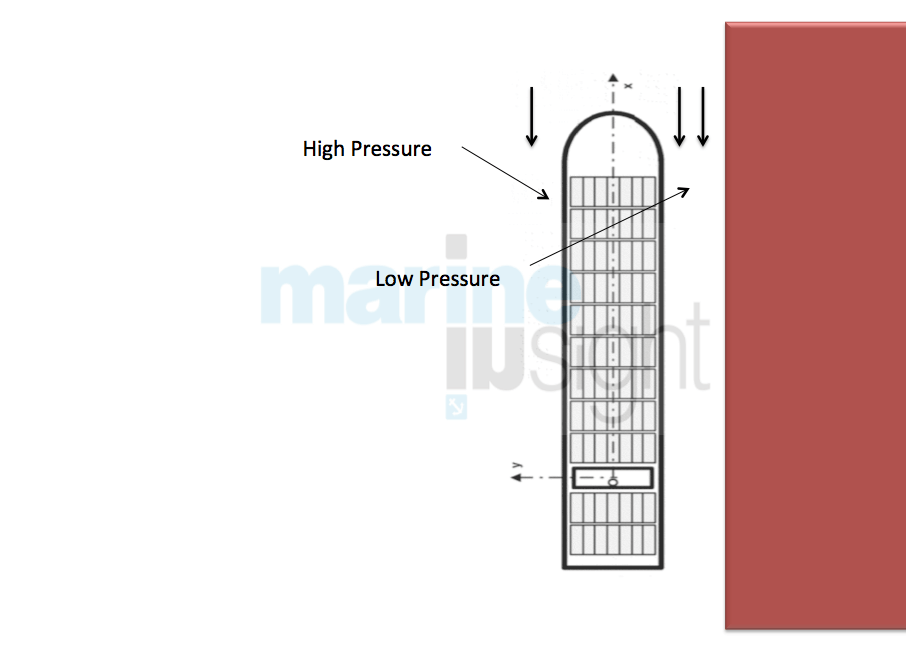
We see the ship is close to the stern on the Starboard side. While the port side is wide open. When the ship plies with considerable speed parallel to the bank, water flow rushing below from the vicinity of the starboard bow towards the stern gets bottled at the constricted space at the stern. But to satisfy the Continuity Equation, its speed increases below the Starboard Quarter. This increase of the speed of the passing water decreases the pressure at the Zs zone than the Zp zone on the Port Quarter.
Consequently water pressure at the port quarter will push the stern more towards the bank making the bow swing towards the center of the channel. This effect of stern moving towards the bank is called the Bank Effect.
A pilot while maneuvering near a bank must slow down to minimize this effect and take the help of rudder to counter it as best as he can. Else he may be forced to move out of the channel. Two ships passing close to each other will experience similar effects in close proximity. Slowing down is the only solution, as usual for both the vessels.
Bank Cushion Effect
When the ship is approaching a steep bank as in fig 4, the water pressure at the starboard bow being less than the port bow ( due the asymmetric flow, explained earlier), the bow is pushed towards the port as to dampen the speed of the fall. This is called Bow cushioning effect.
To minimize these effects the pilot must slow down to avoid any untoward incident or accident.
Over to you..
Have you experienced these forces affecting your ship in restricted waters? Let’s us know about your experiences and what have you done to minimize these effects.
Do you have info to share with us ? Suggest a correction
Latest Marine Navigation Articles You Would Like:
Subscribe To Our Newsletters
By subscribing, you agree to our Privacy Policy and may receive occasional deal communications; you can unsubscribe anytime.















Dear sir, the effects are nicely explained with use of basic physics principles.
Thanks
Very nicely with daigram…
Thanking you..
Beautiful explanations with illustrations that would surely help students of Marine Engineering!
very nicely explained…use of physics basic principle was must and its fusion with ship handling was great
thanx sir eiffect are nicely explained with us along with diagrams. …. its really good to learn this way
If z and z’ be the underwater clearance at fore and aft respectively,(A & B are the underwater clearence).
in the bank cushion effect, if the low pressure is on stbd bow, why is the vessel swinging to port?
Sir, you have made it look very simple & clear, very well explained.
Dear sir,
I have just studied about this problem at my school. After reading this article I have got more detail about this problem and I think this problem have both advantage and disadvantage. Disadvantage is very clearly, but We can use this effect to turn in the channel: When we turn to starboard, we will steer the ship to near the bank in the opposite side combine with the rudder’s force we can turn to stb easy without hard to stb.
Hi,
An excellent article. I do feel another section detailing the classic signs of squat would further amplify this article. As this is a typical Oral’s question and PEC question. I. E. Excessive vibration, double bow wave, bow wave at 90degrees to the bow, tidal wave surge following the ship. Also there is no mention of how the block co-efficient of the ship affects squat. Clearly the higher the block co-efficient the greater the squatting effect.
My ship regularly runs across a shallow channel at high tide and we see all these signs, and naturally have to reduce the pitch to counteract it.
Just my thoughts. Thanks. Hope they can help amplify the article. Cheers
Dan
Gudday,
Thanks for the well written article. My question about the bank cushion effect is that if the low pressure is on the stbd bow, why is the bow taken to port?
Thanks,
Bo
In bow cushion or bank cushion as said here why would bow tend to go to port when the low pressure on starboard side.
Low pressure is negative and our own ships Bow wave is also negative thereby repelling the bank.
hı I need make project dissertation about bank effect .can someone help me ?
A, almost, good and clear explanation. I have a problem with it.
What if a pilot ladder is rigged 2 meter over the waterline when the ship is alongside. When the pilot disembarks the squat is 1 meter, how high over the waterline is the ladder now? If I read the article it is 1 meter. Off course this is wrong. The displacement of the ship is the same as the mass of the ship. Thank you mr. Argimedes. The speed of water does not change this. Wat changes is the speed of the water the higher the speed the lower the pressure. And because the air pressure is the same the ship is moving in a dent in the water. This dent can be observed at passing ships. This dent is the same as the reduction of the under keel clearance . This also is the explanation of the bank effect. On the side of the bank the speed of the water is higher and therefore the level lower as the clear side. This is not a theory but observation and the right way to use the laws of physics .
So the higher the water speed the bigger the dent. Factors like ship speed. The water dept , how wide the channel is and block coefficient ar all factors in water speed around and under the vessel.
Well explained
In my opinion or maybe it is my lack of understanding
Dear Captain – Bank Cushion Effect is not what you have written there.
Bow is pushed away from bank to port by increased pressure between the bow and the bank. Vessel is pushing the water in the front of the bow. When this mass of water get interaction with bank the bow is getting pushed to port.
The other examples are very good. It is good publication.
Dear mr Lukasz,
Not your lack of understanding, indeed the bow wave can push the bow off but the effect of the higher flow rate of the water especially at the stern of a propeller driven vessel induces a water level drop on the “bank side of the vessel” causing the stern to be pushed even closer to the bank. This sucking in effect on the stern ( the water level at the side of the vessel is on the stern higher than at the bow causing the bow to turn out and stern to go in.
thank you for your material to understand to easy.
I think this is a likely contributing factor to the grounding of the Ever Grace….
This article very well explains a complex matter. I have shared the link to a few people (including on Linked in)
Do you think these dynamics affected Ever Given?
Human Error, is a concept of the past and is unsuitable to understand and manage risks of complex and dynamic sociotechnical systems such as shipping.
Professor Jens Rasmussen is clear on this point: ” the explanation of an accident in terms of chains of events, root causes, barriers and human errors is not very useful in improving the safety of a complex and dynamic sociotechnical system.”
Thank you for bringing this up @Hans